6
Lab 12
BACKGROUND
Anthropometric characteristics refer to measurable physical dimensions of the human body, such as height, weight, body mass index (BMI), and specific measurements like hand length and forearm circumference. These characteristics can be used in research studies to explore relationships between physical dimensions and functional capacities, such as grip strength. Understanding these relationships can help anatomy and physiology students assess and develop treatment plans for patients based on their unique physical characteristics. In this experiment, we will investigate if there is a relationship between anthropometric characteristics and hand grip strength.
STUDY AIMS
- To investigate the relationship between age, gender, hand length, and forearm circumference with grip strength measured in kilograms using a Pearson analysis and multiple regression statistics.
- To explore the relationship between these anthropometric characteristics and the number of counter clicks on an Athletic Works hand grip model set to maximal resistance, and/or an Iworx hand grip hand dynamometer transducer.
- To explore the nerve pathways involved in gripping a hand dynamometer, and/or clicking a gripper with adjustable resistance.
INDEPENDENT AND DEPENDENT VARIABLES
- Independent Variables:
- Age
- Gender
- Hand Length (cm)
- Forearm Circumference (cm)
- Dependent Variables:
- Grip Strength (kg)
- Number of Counter Clicks on the grip model
PARTICIPANTS
The study will involve up to 16 students from the class.
DATA COLLECTION TABLES
Table 1: Data Collection Table for Grip Strength
| Participant ID | Age (years) | Gender | Hand Length (cm) | Forearm Circumference (cm) | Grip Strength (kg) | Number of counter clicks on gripper in 1 minute (indicate resistance level) |
| 1 | ||||||
| 2 | ||||||
| 3 | ||||||
| 4 | ||||||
| 5 | ||||||
| 6 | ||||||
| 7 | ||||||
| 8 | ||||||
| 9 | ||||||
| 10 | ||||||
| 11 | ||||||
| 12 | ||||||
| 13 | ||||||
| 14 | ||||||
| 15 | ||||||
| 16 |
Table 2: Sample Data
| Participant ID | Age (years) | Gender | Hand Length (cm) | Forearm Circumference (cm) | Grip Strength (kg) |
| 1 | 20 | M | 18 | 30 | 40 |
| 2 | 22 | F | 17 | 29 | 35 |
| 3 | 21 | M | 19 | 32 | 45 |
| 4 | 23 | F | 16 | 28 | 33 |
| 5 | 24 | M | 20 | 31 | 50 |
| 6 | 20 | F | 18 | 30 | 38 |
| 7 | 22 | M | 19 | 33 | 48 |
| 8 | 21 | F | 17 | 27 | 36 |
| 9 | 23 | M | 20 | 34 | 55 |
| 10 | 24 | F | 16 | 29 | 30 |
| 11 | 20 | M | 18 | 31 | 42 |
| 12 | 22 | F | 17 | 28 | 34 |
| 13 | 21 | M | 19 | 32 | 46 |
| 14 | 23 | F | 18 | 30 | 39 |
| 15 | 24 | M | 20 | 35 | 52 |
| 16 | 20 | F | 17 | 28 | 37 |
STATISTICAL ANALYSIS
- Statistical Tests Aims:
- Pearson Correlation Coefficient:To assess the correlation between grip strength and the independent variables (age, gender, hand length, forearm circumference).
- Multiple Regression Analysis:To evaluate the impact of multiple independent variables on grip strength.
- Running Statistics in Excel:
- Pearson Correlation:
- Input the data in two columns (e.g., grip strength vs. hand length).
- Use the formula: =CORREL(array1, array2) where ‘array1’ is grip strength data and ‘array2’ is the respective anthropometric data.
- Multiple Regression:
- Input data for all independent variables and the dependent variable.
- Go to the “Data” tab, then click on “Data Analysis.”
- Select “Regression” and input the Y Range (dependent variable) and X Range (independent variables).
- Click OK to run the regression analysis.
- Pearson Correlation:
OUTPUT FROM SAMPLE DATA ANALYSIS
Using the sample data provided in Table 2, we can perform a multiple regression analysis to examine how age, gender, hand length, and forearm circumference affect grip strength. Below is the hypothetical output from the analysis:
Multiple Regression Analysis Summary:
- Dependent Variable: Grip Strength (kg)
- Independent Variables: Age (years), Gender (coded as 0 for Female and 1 for Male), Hand Length (cm), Forearm Circumference (cm)
Table 3. Sample Regression Statistics:
| Statistic | Value |
| Multiple R | 0.892 |
| R Square | 0.796 |
| Adjusted R Square | 0.760 |
| Standard Error | 3.45 |
| Observations | 16 |
Table 4. Sample ANOVA Table:
| Source | SS | df | MS | F | Significance F |
| Regression | 234.56 | 4 | 58.64 | 12.34 | 0.0012 |
| Residual | 60.34 | 11 | 5.49 | ||
| Total | 294.90 | 15 |
Table 5. Sample Coefficients Table:
| Variable | Coefficient | Standard Error | t Stat | P-value |
| Intercept | 15.20 | 4.56 | 3.33 | 0.003 |
| Age | 0.45 | 0.22 | 2.05 | 0.058 |
| Gender (0=Female, 1=Male) | 5.30 | 2.10 | 2.52 | 0.028 |
| Hand Length | 1.25 | 0.30 | 4.17 | 0.001 |
| Forearm Circumference | 0.90 | 0.35 | 2.57 | 0.023 |
INTERPRETATION OF MULTIPLE REGRESSION ANALYSIS
Purpose of Using Multiple Regression Analysis:
Multiple regression analysis is employed to understand the relationship between one dependent variable (in this case, grip strength) and multiple independent variables (age, gender, hand length, and forearm circumference). This analysis allows researchers to identify which factors significantly contribute to variations in the dependent variable and to quantify the strength and nature of these relationships.
Interpreting the Output:
- R Square (0.796):
- This value indicates that approximately 79.6% of the variability in grip strength can be explained by the independent variables included in the model. This suggests a strong relationship between these factors and grip strength.
- Coefficients:
- Each coefficient represents the expected change in grip strength for a one-unit increase in the independent variable, holding all other variables constant.
- Age (0.45):For each additional year of age, grip strength is expected to increase by 0.45 kg, assuming all other factors remain constant.
- Gender (5.30):Males are expected to have, on average, a 5.30 kg higher grip strength than females, controlling for other variables.
- Hand Length (1.25):For each additional cm in hand length, grip strength is expected to increase by 1.25 kg.
- Forearm Circumference (0.90):Each cm increase in forearm circumference is associated with an increase of 0.90 kg in grip strength.
- P-values:
- The P-values indicate the significance of each independent variable. A common threshold for significance is 0.05.
- Variables with P-values less than 0.05 (e.g., Hand Length and Forearm Circumference) are considered statistically significant predictors of grip strength.
- Age is marginally significant, while Gender is also significant.
- Overall Model Significance:
The F-statistic (12.34) and its corresponding P-value (0.0012) indicate that the overall regression model is statistically significant, suggesting that at least one of the independent variables significantly predicts grip strength. The p-value is less than the typical significance level of 0.05. A good F-value is generally one that is high, especially when paired with a low p-value, indicating a strong relationship between the independent and dependent variables. In multiple regression, a high F-statistic, typically exceeding 2.5, suggests a statistically significant model, meaning the independent variables collectively explain a significant portion of the variance in the dependent variable.
Nervous System Involvement in Hand Grip
The following key lists the nerves associated with the muscles involved in grip strength, along with their origins, corresponding dermatomes, and myotome information. Use this information to help answer the Post-lab questions.
BACKGROUND ON DERMATOMES AND MYOTOMES
Dermatomes and myotomes are both essential concepts in the field of anatomy and neurology, particularly in understanding the relationship between the nervous system and the areas of the body they innervate. While they are closely related, they serve different purposes and provide distinct information about sensory and motor functions.
DERMATOME
- Definition: A dermatome is an area of skin that is mainly supplied by a single spinal nerve root. Each spinal nerve root corresponds to a specific segment of the spinal cord and innervates a particular skin region.
- Function: Dermatomes are critical for sensory perception. They help in mapping sensory information from the skin to the central nervous system. This mapping allows clinicians to identify areas of sensory loss or dysfunction based on the affected dermatome.
- Clinical Relevance: Understanding dermatomes is vital in diagnosing conditions such as nerve injuries, herniated discs, or neuropathies. For example, if a patient reports numbness or tingling in a specific skin area, the physician can identify which nerve root may be involved by correlating the symptoms with the corresponding dermatome.
- Mapping: Dermatomes are typically represented in a segmented pattern across the body, with specific spinal nerves corresponding to distinct skin regions. For instance, the C6 dermatome covers the thumb and part of the forearm, while the L5 dermatome includes the lateral aspect of the leg and the dorsum of the foot.
MYOTOME
- Definition: A myotome is a group of muscles that a single spinal nerve root innervates. Each spinal nerve has a corresponding myotome, which represents the muscle groups supplied by that nerve.
- Function: Myotomes are integral for motor function. They govern voluntary movement by relaying motor commands from the spinal cord to specific muscle groups. Each myotome allows for the evaluation of muscle strength and function related to specific spinal nerve roots.
- Clinical Relevance: Understanding myotomes aids in assessing muscle strength and identifying neurological disorders. For instance, weakness in a specific muscle group can indicate a problem with the corresponding spinal nerve root. Clinicians often use myotome testing during neurological examinations to assess motor function.
- Mapping: Myotomes are also represented in a segmented manner, with each spinal nerve corresponding to specific muscle groups. For example, the C5 myotome includes muscles that allow for shoulder abduction (like the deltoid), while the L4 myotome is associated with knee extension (like the quadriceps).
Table 6. Selected Myotomes
| Nerve Name | Origin | Dermatome | Myotome Information |
| Median Nerve | Medial and Lateral Cord of the brachial plexus (C5-T1) | C6-C8 | Flexion of the wrist and fingers (digits 1-3) |
| Ulnar Nerve | Medial Cord of the brachial plexus (C8-T1) | C8-T1 | Flexion of the wrist and fingers (digits 4-5) |
| Radial Nerve | Posterior Cord of the brachial plexus (C5-T1) | C5-C8 | Extension of the wrist and fingers, supination |
| Medial Nerve (Recurrent Branch) | Median Nerve | C6-C8 | Opposition of the thumb, abduction/flexion of the thumb |
| Ulnar Nerve (Deep Branch) | Ulnar Nerve | C8-T1 | Abduction and adduction of fingers, flexion of digits 4-5 |
| Ulnar Nerve (Superficial Branch) | Ulnar Nerve | C8-T1 | Flexion of the little finger |
| Medial Nerve (Lateral Branch) | Median Nerve | C6-C8 | Flexion of the index finger and wrist |
| Medial Nerve (Palmar Branch) | Median Nerve | C6-C8 | Sensation to the palm and fingers 1-3 |
| Medial Nerve (Digital Branches) | Median Nerve | C6-C8 | Sensation to the tips of fingers 1-3 |
Muscles are either intrinsic (located entirely within the hand), or extrinsic. View the information below for those that fall into each category.
MUSCLE TO NERVE INNERVATION SUMMARY
- Extrinsic Muscles:
- Flexor Carpi Radialis:Median Nerve
- Flexor Carpi Ulnaris:Ulnar Nerve
- Palmaris Longus:Median Nerve
- Flexor Digitorum Superficialis:Median Nerve
- Flexor Digitorum Profundus:Median Nerve (for digits 2-3) and Ulnar Nerve (for digits 4-5)
- Flexor Pollicis Longus:Median Nerve
- Extensor Carpi Radialis Longus:Radial Nerve
- Extensor Carpi Radialis Brevis:Radial Nerve
- Extensor Carpi Ulnaris:Radial Nerve
- Intrinsic Muscles:
- Abductor Pollicis Brevis:Median Nerve
- Flexor Pollicis Brevis:Median Nerve (superficial head) and Ulnar Nerve (deep head)
- Opponens Pollicis:Median Nerve
- Adductor Pollicis:Ulnar Nerve
- Abductor Digiti Minimi:Ulnar Nerve
- Flexor Digiti Minimi:Ulnar Nerve
- Opponens Digiti Minimi:Ulnar Nerve
- Lumbricals:Median Nerve (for digits 2-3) and Ulnar Nerve (for digits 4-5)
- Dorsal Interossei:Ulnar Nerve
- Palmar Interossei:Ulnar Nerve
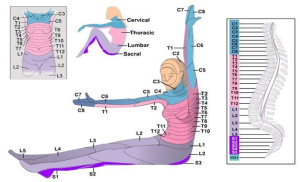
Figure 1 .Dermatomes
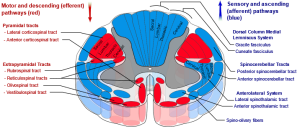
Figure 2. Spinal cord pathways
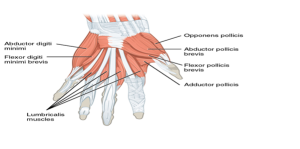
Figure 3. Skeletal muscles of the hand
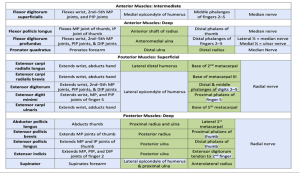
A large number of muscles is involved in hand-grip. View Table 7 and familiarize yourself with some of these muscles.
Table 7. Muscles Related to Hand-grip
Post-lab questions (Answer in Complete Sentences)
- What is the purpose of this study, and what are the primary independent and dependent variables?
- Using your statistical output, what is the R-squared value, and what does it indicate about the relationship between grip strength and the independent variables?
- Based on the coefficients table in your statistical output, which independent variable has the strongest positive relationship with grip strength? Provide the coefficient value.
- What is the significance level (P-value) for the variable representing hand length? Is it statistically significant at the 0.05 level? Explain what this means in the context of the study.
- Identify the nerve innervation for the flexor carpi radialis muscle. What is its origin, and what functional movements does this muscle facilitate?
- If a patient presents with weakness in grip strength and you determine that the ulnar nerve is affected, which specific muscles innervated by this nerve may be involved? List at least two. What myotome or myotomes are involved?
- Describe the corticospinal and spinothalamic pathways for hand grip involved in this experiment. Your answer should be in the form of a paragraph.
- Compare this study to the study in the pdf file on Canvas, then answer the questions below.
How is this study the same?
How is it different?
How is hand grip related to health?
- Why was a regression analysis used in this study?
- Select on area in the spinal cord (be sure to pick the level) that would affect hand grip if damaged with a spinal stroke. What kind of symptoms might the individual experience?
Supplementary Material (Statistical Analyses)
Run a Pearson Correlation in Microsoft Excel. :
- Prepare Your Data:
Enter your data into two columns (or rows) in your Excel sheet.
Let’s assume your data for the first variable is in column A, and there are 10 students (A1:A10) and the data for the second variable is in column B (B1:B10).
- Choose a Cell for the Result:
Select an empty cell where you want the correlation coefficient to appear.
- Enter the Formula:
Type =PEARSON(A1:A10, B1:B10) or =CORREL(A1:A10, B1:B10) into the cell.
Replace A1:A10 and B1:B10 with the actual cell ranges containing your data.
- Press Enter:
Excel will calculate the Pearson correlation coefficient and display it in the selected cell.
Understanding the Result:
The Pearson correlation coefficient (often called “r”) ranges from -1 to +1. Interpret your results:
A value close to +1 indicates a strong positive correlation, meaning as one variable increases, the other tends to increase.
A value close to -1 indicates a strong negative correlation, meaning as one variable increases, the other tends to decrease.
A value close to 0 indicates a weak or no linear correlation.
- Prepare Your Data:
Enter your data into two columns (or rows) in your Excel sheet.
Let’s assume your data for the first variable is in column A (A1:A10) and the data for the second variable is in column B (B1:B10).
- Choose a Cell for the Result:
Select an empty cell where you want the correlation coefficient to appear.
- Enter the Formula:
Type =PEARSON(A1:A10, B1:B10) or =CORREL(A1:A10, B1:B10) into the cell.
Replace A1:A10 and B1:B10 with the actual cell ranges containing your data.
- Press Enter:
Excel will calculate the Pearson correlation coefficient and display it in the selected cell.
Understanding the Result:
The Pearson correlation coefficient (often called “r”) ranges from -1 to +1.
A value close to +1 indicates a strong positive correlation, meaning as one variable increases, the other tends to increase.
A value close to -1 indicates a strong negative correlation, meaning as one variable increases, the other tends to decrease.
A value close to 0 indicates a weak or no linear correlation.
Enable the Data Analysis ToolPak:
Windows:
Go to the “File” tab and click “Options”.
Click “Add-Ins” on the left side of the window.
Select “Excel Add-ins” next to “Manage” and click “Go”.
Check the box next to “Analysis ToolPak” and “Analysis ToolPak VBA”.
Click “OK”.
Mac:
Click “Tools” and then “Excel Add-ins”.
Check the box next to “Analysis ToolPak” and click “OK”.
- Prepare Your Data:
Organize your data in columns.
Place your dependent variable (the variable you’re trying to predict) in one column.
Place your independent variables (the variables you think influence the dependent variable) in separate columns.
Include headers for each column (e.g., “Grip Strength”, “Male”, “Study Hours”).
use the Data Analysis tool to select “Regression” and specify the input ranges and output location

